As I was trying to understand the Whitehead manifold and related constructions of non-tame manifolds batter, I guess it makes a cool blog post ^^
The Whitehead manifold is an example of a 3-manifold that’s contractable but not homeomorphic to i.e. the manifold is homotopically equivalent to a point but not tame. (See the earlier post on tameness for more explanations)
Construction:
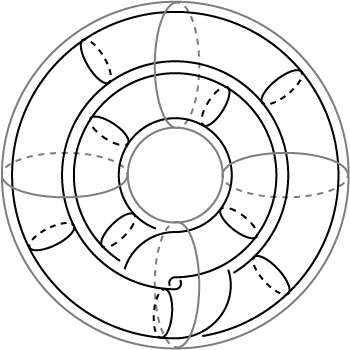
Take a solid torus , embed a thinner torus
as shown:
Iterate the process: at each step, embed solid torus into
so that
“links with itself” inside
:
Let the Whitehead continuum be the intersection of the s.
i.e.
As some people know, I have a weird hobby of describing strange continua in terms of Cantor sets…So here comes ‘Conan’s translation’ of the Whitehead continuum:
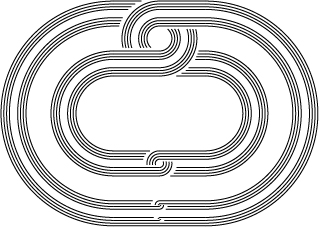
Take two copies of where
is the standard middle-third Cantor set. Bend them into Rainbow-shape with the open ends facing each other:
(I think of this as having a width ‘brush’ with ink only on the points of the Cantor set, and use the brush to draw two semicircles)
Now we connect the open ends: take a width brush and connect the top pair of ends so that they link with each other, and then a width
brush for the highest remaining pair, etc. Take the union of all those connecting sets, union a line segment joining the bottom-most pair of points, we get the Whitehead continuum:
The Whitehead manifold is the complement of in the three-sphere
, equipped with the
What’s the fundamental group of the Whitehead manifold?
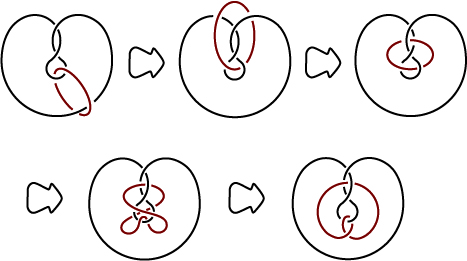
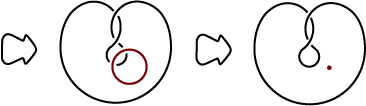
Claim: is null-homotopic in
.
pf: is contractible to a loop in
, hence it suffice to homotope the red loop to a point without touching the black loop:
Note that for homotopy, the loop is allowed to pass through itself: (in contrast to isotopy)
The loop can now be easily contracted:
Hence we deduce the Whitehead manifold is null-homotopic. (by collapsing each at some finite time)
In particular, it has trivial fundamental group! (This might seem hard to believe especially when looking at my Cantor-set picture) Infact for this, we can directly see from the picture that all loop can be homotoped to constant:
Since loop is compact, there is a ‘finest gap’ in the Cantor set which the loop passes through, say it’s a gap with width . Now by performing the operation above, we can homotope all parts of the loop that goes through the
to segments that goes through
-gaps, by having the segments crossing themselves once. Now we pass to the
-gaps, etc. until the loop lie completely outside the thickened disc which the continua lies in. Once it’s outside the disc, the loop can be contracted.
The manifold is not homeomorphic to as we can easily see that, unlike in
, the red loop in picture cannot be isotoped to a trivial loop.
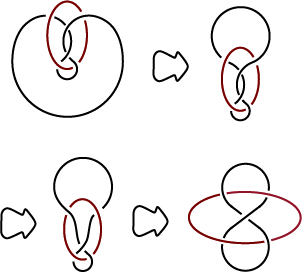
As an alternative point of view, we should note that in fact and
form a thickened Whitehead link:
Since the Whitehead link is symmetric, this gives an simpler (but less direct, in my opinion) way of knowing that red loop is homotopically trivial in the complement of the black loop. (As the black loop is obviously homotopically trivial in the complement of the red loop.)
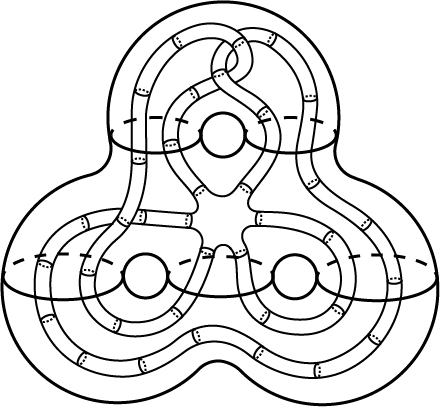
In light of this, one may construct many different non-tame manifolds with finitely generated fundamental group by embedding a handlebody inside another copy of itself and take the complement of the infinite intersection.
Here is an example of embedding a genus handlebody. The resulting manifold (after taking the complement of the intersection) is a homotopy genus
handlebody. (As in the whitehead case,
can be homotoped to a genus
handlebody inside
. The ‘third loop’ can be unknotted by crossing itself once.) But it’s of coruse not homeomorphic to the genus
handlebody.


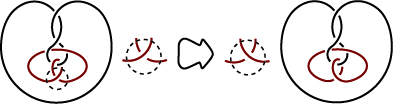
You Cantormania….
LikeLike
[…] called the Whitehead manifold. While asking Google to tell me the Wikipedia link again I noticed this post that appears to give a decent overview of the Whitehead […]
LikeLike
Loved this post, helpful when reading this paper. I might make a similar post and link to you
LikeLike