Tag: digital painting

Back to the drawing board — Month #1 (and a bit more)

Conan3 Comments

So this is the first post after my blog (and life) transition! Just wish to record some progress in my concept art studies after not drawing and painting for 6 years. Feel so good that sometimes I can’t believe this is going to be what I do full-time! (Why didn’t I start this 1.5 years ago?!)

In any case, the goal of this blog is to have bad paintings and drawings at the beginning and hopefully show improvements over time. My ultimate hope is for this to envolve into a log that shows dreams can be achieved no matter how distant it is from where you are.

Anyways, here are some selected pages of what I’ve been doing over the last month or so =P

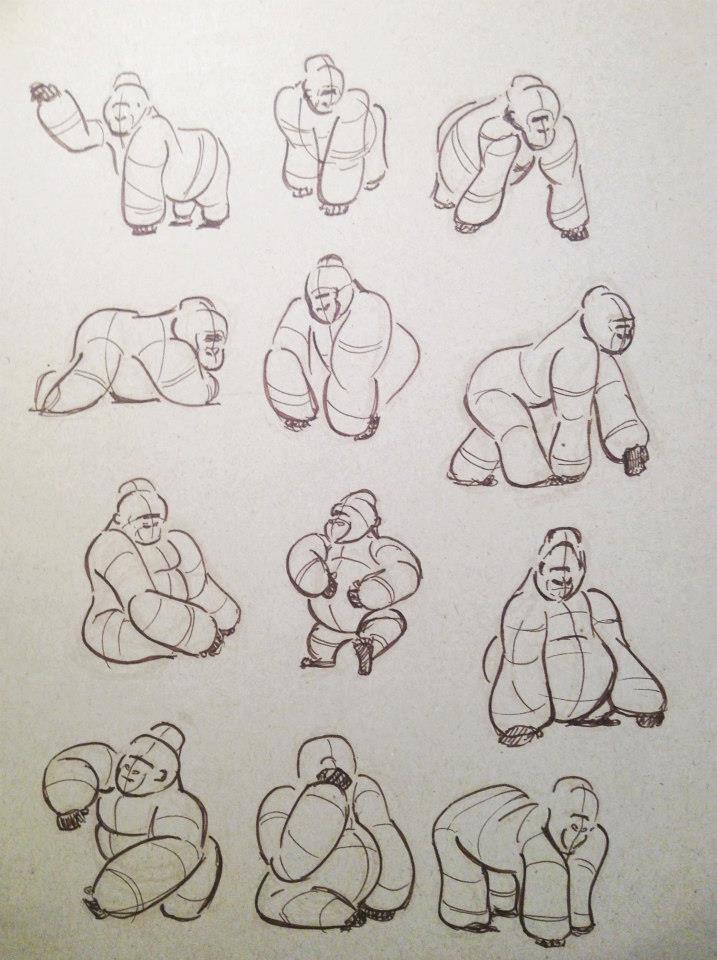
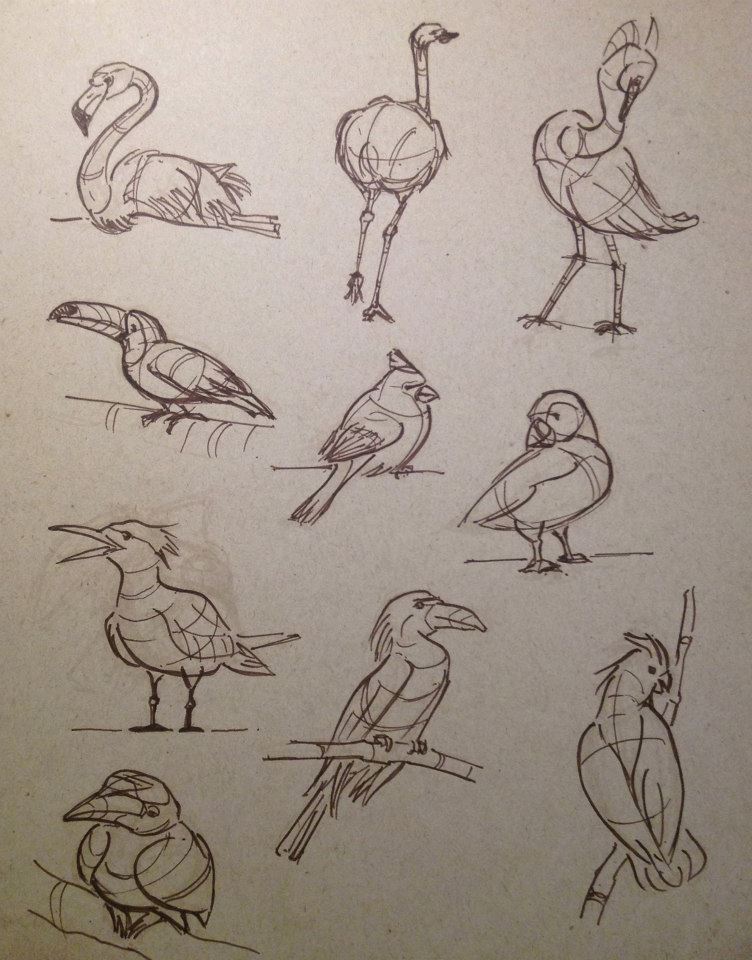
First, some animal thumbnails~ (went to the zoo last week, but those are mostly from photo as I’m still not very comfortable drawing while standing in the crowd >.<)

1002780_4894076963530_1093662084_n

Gorillas are super fun to draw!
5

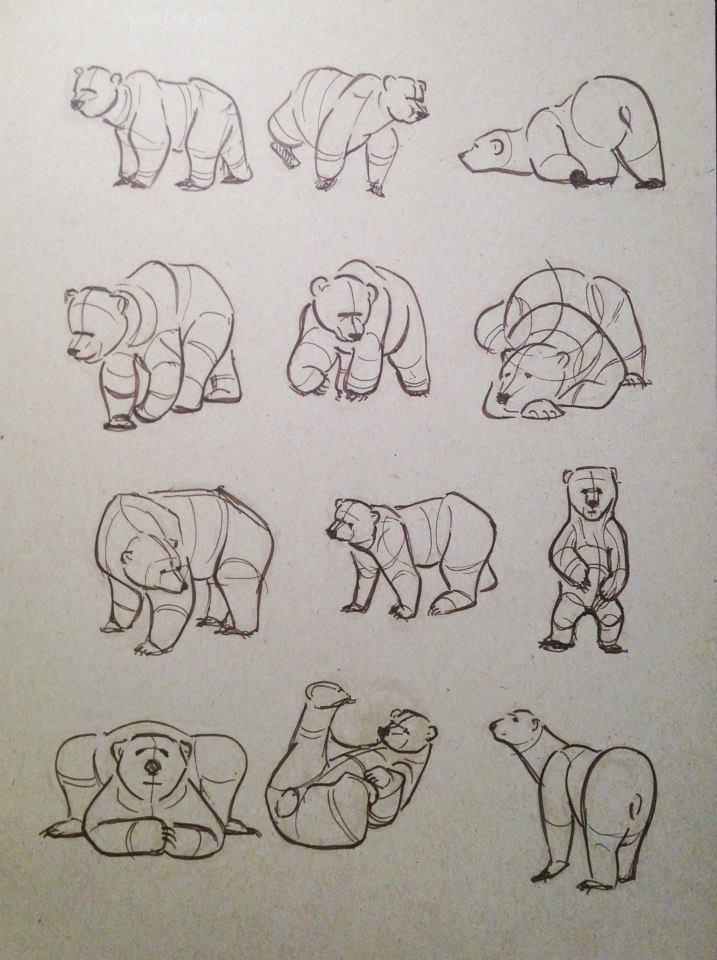
Bears (and some polar bears)

2

African collection

3

Birds

26

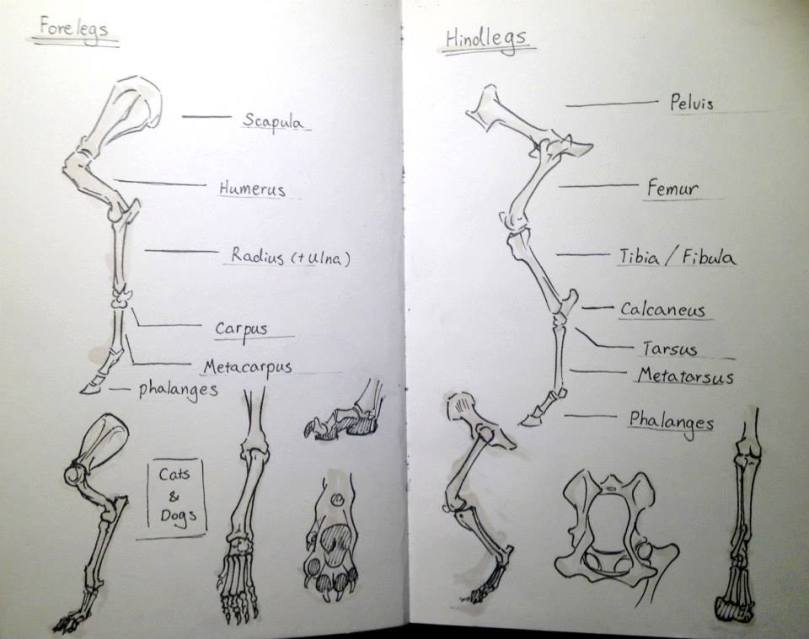
This is me trying to figure out how does animal legs bend…

6

Copied animal poses before the zoo, to get a better idea of how to capture the gesture.

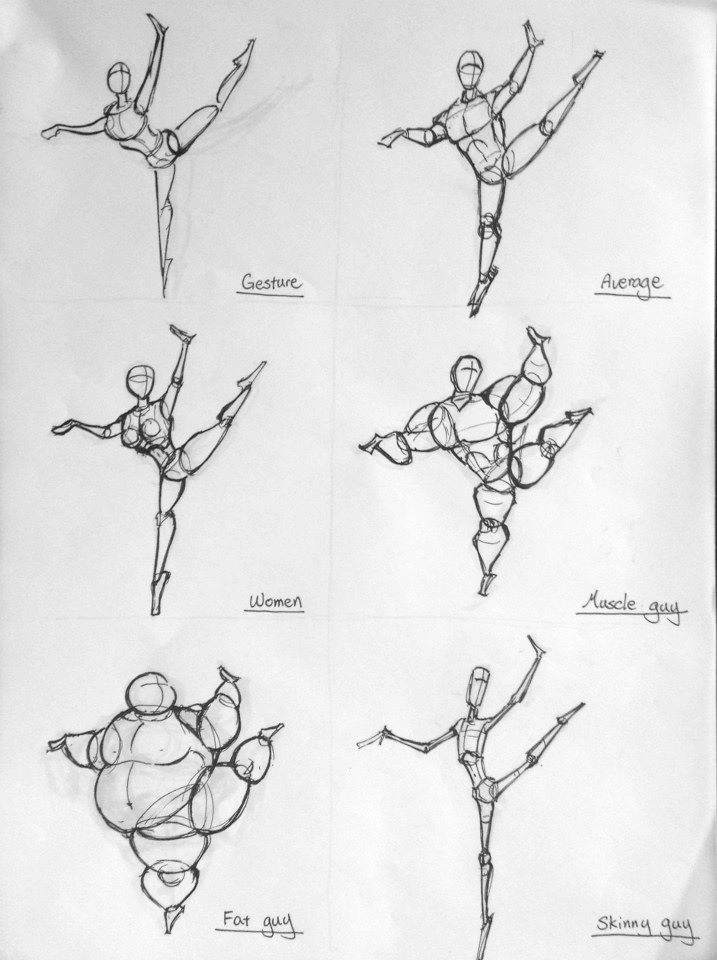
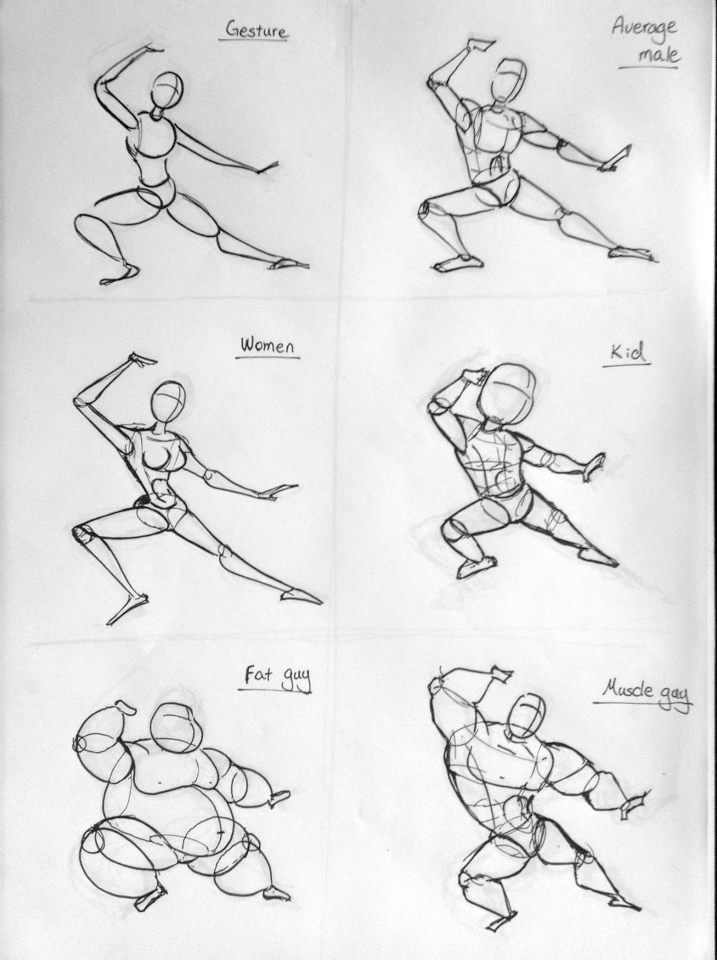
Now onto human figure invention~

7

Those are given a background and invent a character to put into the scene.

8

This was super fun to draw~ I am happy to see that now I can draw figures without finding a photo reference ^^

11

Take a pose and change the character posing. (we actually had a model doing in and was asked to change him into ‘superhero’, ‘sexy women’ etc =P)

1-

I think I really like drawing fat guys…

1817

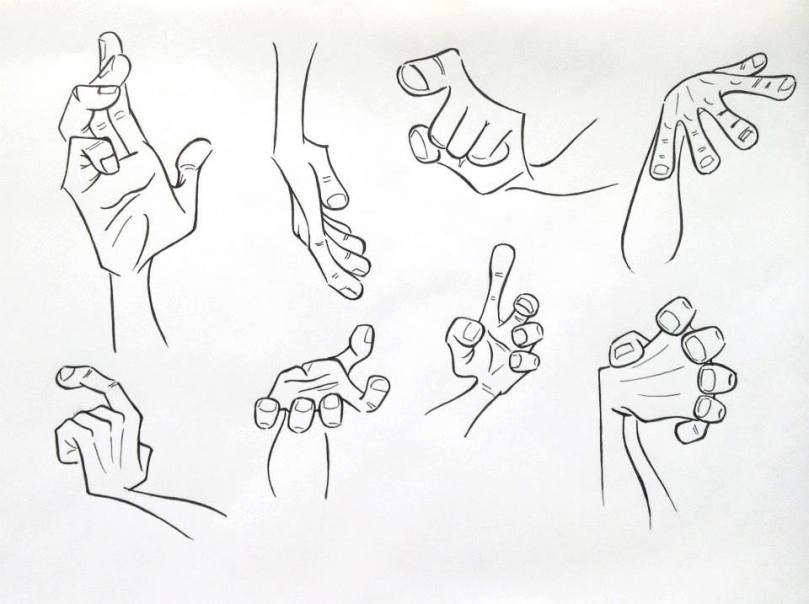
Hand expressions, roughly a half of them copied from master animators from Disney, the other half drawn according to my own (left) hand =P

24

Designing a ‘space pirate’.

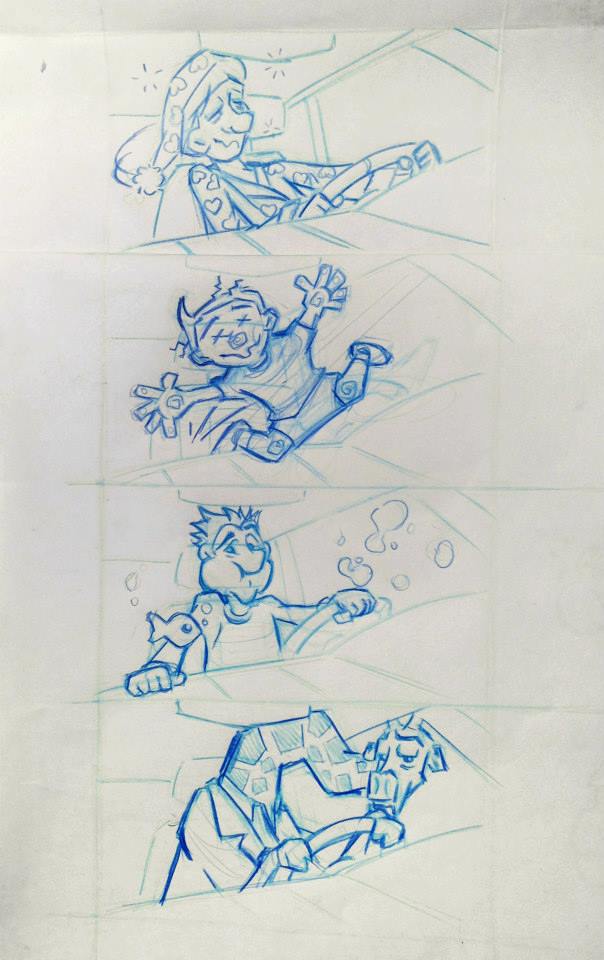
Okay, below are some absolutely uncategorized random stuff I just decided to throw in:
1

A little layout. (obviously inspired by Ratatouille)

23

Some plants (drawn on spot in Caltech)

25

Balls, painted in Photoshop

Okay…onto…oil painting! I have to say that I have never done oil in my life…(and I only ever painted a couple times back in high school >.<) But it’s so exciting to start!!!

I started off by going to those life model sessions where there is a model posing and everyone drops in and paints:

13

15

22

14

12

First-time still life.

20
21

Some thumbnail landscapes from my travel photos.

Anyways, painting is something I am most looking forward to improve! Just started to systematically learning it last week, so stay turned!

A sketch of a piece I really wanted to paint once I get better at it:

9

A train track on twice punctured torus

Conan4 Comments

This is a non-technical post about how I started off trying to prove a lemma and ended up painting this:

One of my favorite books of all time is Thurston‘s ‘Geometry and Topology of 3-manifolds‘ (and I just can’t resist to add here, Thurston, who happen to be my academic grandfather, is in my taste simply the coolest mathematician on earth!) Anyways, for those of you who aren’t topologists, the book is online and I have also blogged about bits and parts of it in some old posts such as this one.

I still vividly remember the time I got my hands on that book for the first time (in fact I had the rare privilege of reading it from an original physical copy of this never-actually-published book, it was a copy on Amie‘s bookshelf, which she ‘robbed’ from Benson Farb, who got it from being a student of Thurston’s here at Princeton years ago). Anyways, the book was darn exciting and inspiring; not only in its wonderful rich mathematical content but also in its humorous, unserious attitude — the book is, in my opinion, not an general-audience expository book, but yet it reads as if one is playing around just to find out how things work, much like what kids do.

To give a taste of what I’m talking about, one of the tiny details which totally caught my heart is this page (I can’t help smiling each time when flipping through the book and seeing the page, and oh it still haunts me >.<):

This was from the chapter about Kleinian groups, when the term ‘train-track’ was first defined, he drew this image of a train(!) on moving on the train tracks, even have smoke steaming out of the engine:

To me such things are simply hilarious (in the most delightful way).

Many years passed and I actually got a bit more into this lamination and train track business. When Dave asked me to ‘draw your favorite maximal train track and test your tube lemma for non-uniquely ergodic laminations’ last week, I ended up drawing:

Here it is, a picture of my favorite maximal train track, on the twice punctured torus~! (Click for larger image)

Indeed, the train is coming with steam~

Since we are at it, let me say a few words about what train tracks are and what they are good for:

A train track (on a surface) is, just as one might expect, a bunch of branches (line segments) with ‘switches’, i.e. whenever multiple branches meet, they must all be tangent at the intersecting point, with at least one branch in each of the two directions. By slightly moving the switches along the track it’s easy to see that generic train track has only switches with one branch on one side and two branches on the other.

On a hyperbolic surface S_{g,p}, a train track is maximal if its completementry region is a disjoint union of triangles and once punctured monogons. i.e. if we try to add more branches to a maximal track, the new branch will be redundant in the sense that it’s merely a translate of some existing branch.

As briefly mentioned in this post, train tracks give natural coordinate system for laminations just like counting how many times a closed geodesic intersect a pair of pants decomposition. To be slightly more precise, any lamination can be pushed into some maximal train track (although not unique), once it’s in the track, any laminations that’s Hausdorff close to it can be pushed into the same track. Hence given a maximal train track, the set of all measured laminations carried by the train track form an open set in the lamination space, (with some work) we can see that as measured lamination they are uniquely determined by the transversal measure at each branch of the track. Hence giving a coordinate system on \mathcal{ML})(S).

Different maximal tracks are of course them pasted together along non-maximal tracks which parametrize a subspace of \mathcal{ML}(S) of lower dimension.

To know more about train tracks and laminations, I highly recommend going through the second part of Chapter 8 of Thurston’s book. I also mentioned them for giving coordinate system on the measured lamination space in the last post.

In any case I shall stop getting into the topology now, otherwise it may seem like the post is here to give exposition to the subject while it’s actually here to remind myself of never losing the Thurston type childlike wonder and imagination (which I found strikingly larking in contemporary practice of mathematics).

Back to the drawing (painting) board

Conan1 Comment

OK, so recently I made some attempts in painting on computer (meaning open a blank canvas in Photoshop and paint on a tablet). After spending just a few nights on it, I can’t help wondering ‘why haven’t I done this earlier?!’. Anyways, to help saving fellow mathematicians from making the same mistake, I’m writing this post. (okay you got me, I just want to post because I think it’s cool >..< Oh well, I guess travelling salesman has to wait q few days.)

This one was painted last night (you should blame it if you can’t wait to read about the travelling salesman problem). After reviewing pervious attempts, I found somehow I tend to use less saturated colors when shifting from canvas to digital. Hence I picked a circus scene for color training (the scene was from Disney’s Hunchback of Notre Dame album), seem to work~ Also, before this I have been sketching at a smaller size hence details a little smoother on this one, click to enlarge.

OK, here comes my complete digital painting history to date:

The very first sketch I did the night my tablet arrived (by the way, I’m using a Wacom Intuos, small). This is a scene I saw back in Evanston, when I used to take mid-night long walks along the shore of lake Michigan, there were days when moon rise from the lake, creating a shiny silver region that silently sparkles in the mideast of the otherwise dark water. My picture certainly doesn’t do the justice, but this was one of the views I have been longing to capture but can’t do with a camera. (low light + moving water) People, if you every visit Northwestern, I highly recommend checking the moonrise schedule!

After the first try (which is mainly black and white), I decided that I should get familiar with color mixing techniques, since the eye-dropper tool is non-existant in the real world. So I did a ‘master study’ i.e. pulled out a hard copy of Pixar concept drawing (originally in colored chalk I believe) and tried to get all the colors working ^_^ So here comes my version of radiator springs from Cars~

The next day I went out of my office, sat on the stairs for a couple hour with a computer and tablet, did this quick sketch of “the only interesting piece of architecture around the math department” – Lewis library by Frank Gary. (never liked the green and orange blocks on the building hence I intentionally deleted them :-P) Can’t get into any details because it was past midnight and that day was fairly cold.

In conclusion, digital vs. traditional:

Digital:
1. Much better at anything to do with color (mixing, getting color from another part of the picture, etc);
2. Faster (used to take me weeks to finish a painting)
3. Unlimited resources (creating custom brushes, color won’t run out)

Traditional:
1. More personal satisfactory (well, I guess nothing compares to holding canvas in you hands)
2. Can actually see the brush and stroke at the same time (took me some time to get used to not seeing where my hands are, but in fact not as bad as one might think)

Anyways, I’m about a week old in this field, so don’t believe anything I said :-P. By the way, a side effect of doing this is, I have now started to think about the RGB value each time I see a colored object in real life >.<.

Hope you had fun! (I certainly did)